Professional Learning
XPDitions Journeys into Professional Development
Teacher learning experiences that deepen content knowledge, expand the repertoire of instructional strategies, emphasize struggling learners’ support, and build a network.
Critical features of PD
The critical features of our professional development associated with x to Why and, most importantly, MPower, define the dimensions of what should be included in any type of delivery. These features are linked to important outcomes that should be observed in classroom practice. They are based on results that were gathered from professional development offerings in the Hawaii Algebra Learning Project (Dougherty, Zenigami, & Matsumoto, 1999) and other associated projects such as Project DELTA (Dougherty, PI, 2008). In the former, changes were measured in changes in teachers’ pedagogical approach to teaching algebra as determined by observational data (established baseline and multiple observations in the following two years) and changes in student performance on a standardized assessment. In the latter, the results were based on multiple criteria: 1) changes in teacher content knowledge and pedagogical content knowledge as determined by the DTAMS (Bush et al., 2007); 2) comparison of teachers’ work samples taken from a baseline task to and ending task; 3) changes in student scores on state assessment; and 4) changes in criteria teachers used in examining student work.
Given the results obtained from previous projects, four critical features of the PD were identified that are unique. These four include the following.
1. The professional development is constructed to provide teachers with experiences on a continuum.
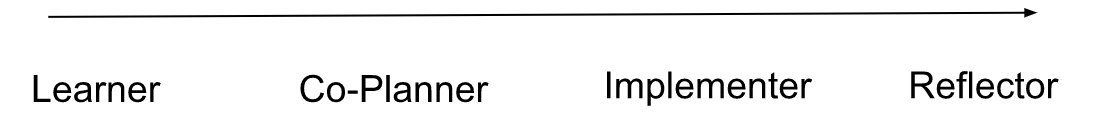
In the Learner stage, participants engage in mathematical tasks that embody significant design features. Even though the tasks may be at, say, a middle school level, they are slightly altered to provide teachers ‘real’ mathematical experiences at a higher level, causing them to rethink their understandings. The instructional model used by the PD instructor mirrors the strategies that would be used in a middle or secondary mathematics class, including a debriefing on the task’s solution and solution process. Thus, teachers gain an understanding of what the learner experiences.
In the Co-planner stage, the debriefing in the Learner stage shifts to a focus on the instruction and classroom implementation. The PD instructor can respond to participants’ questions about how the task and its associated instruction can be implemented in a classroom. Teachers think about how they might implement the task and strategies in their own class while they have the support of the instructor and other participants. At this stage, criteria for determining the success or effectiveness is considered.
In the Implementer stage, participants implement the task in some form. It may also be the case that they choose to implement an instructional strategy with another task. Regardless, they try something that is different than their ‘normal’ instruction. The PD instructor may observe the lesson ‘live,’ watch a video of the lesson, or review and analyze student work or other data with the teacher. Teachers may also request that the instructor co-teach a selected lesson with the next lesson taught by the teacher with the PD instructor observing.
In the final stage, participants reflect on the implementation, evaluate the results, and consider what they will do next. The success criteria identified during the co-planning stage are considered and applied to l determine the success or effectiveness of what is implemented. The PD instructor’s role can be as a coach or a critical peer or an objective voice, asking clarifying questions or co-planning next steps.
2. The instructor models effective questioning.
The mathematics group at CRDG has at its core a strong questioning framework. While the tasks are designed with these questions embedded, the discussion that follows the participants engaging in a task is led by the instructor who models follow-up questions. This demonstrates for participants how the student discourse fuels diving into deeper mathematics. Generalizations may be made and misconceptions addressed in ways that are different from a traditional didactic approach.
3. The instructor models effective instructional strategies to promote student engagement.
The mathematics group developed grouping techniques that foster increased student engagement in solving tasks and debriefing or discussing mathematical ideas. The grouping structures move from individual work to dyads, then to small groups, further to combinations of small groups, and finally public or whole class techniques.
In addition to grouping techniques, more implicit strategies are used, including, but not limited to, the following:
- Archiving student responses by scripting
- Cuing groups with additional questions specific to that group’s engagement and discussion
- Organizing public discussions so that there is a purposeful sequence to solution methods being presented
4. Participants analyze mathematics tasks.
Mathematics tasks from participants’ materials or from PD materials are analyzed to determine barriers or challenges students may face. The analysis also focuses on the mathematical ideas that are built from the task and may not be evident by looking only at the task as it is presented to students.
